Order 21 Perfect Square Puzzle | Mathematical Brain Teaser
Description
Description
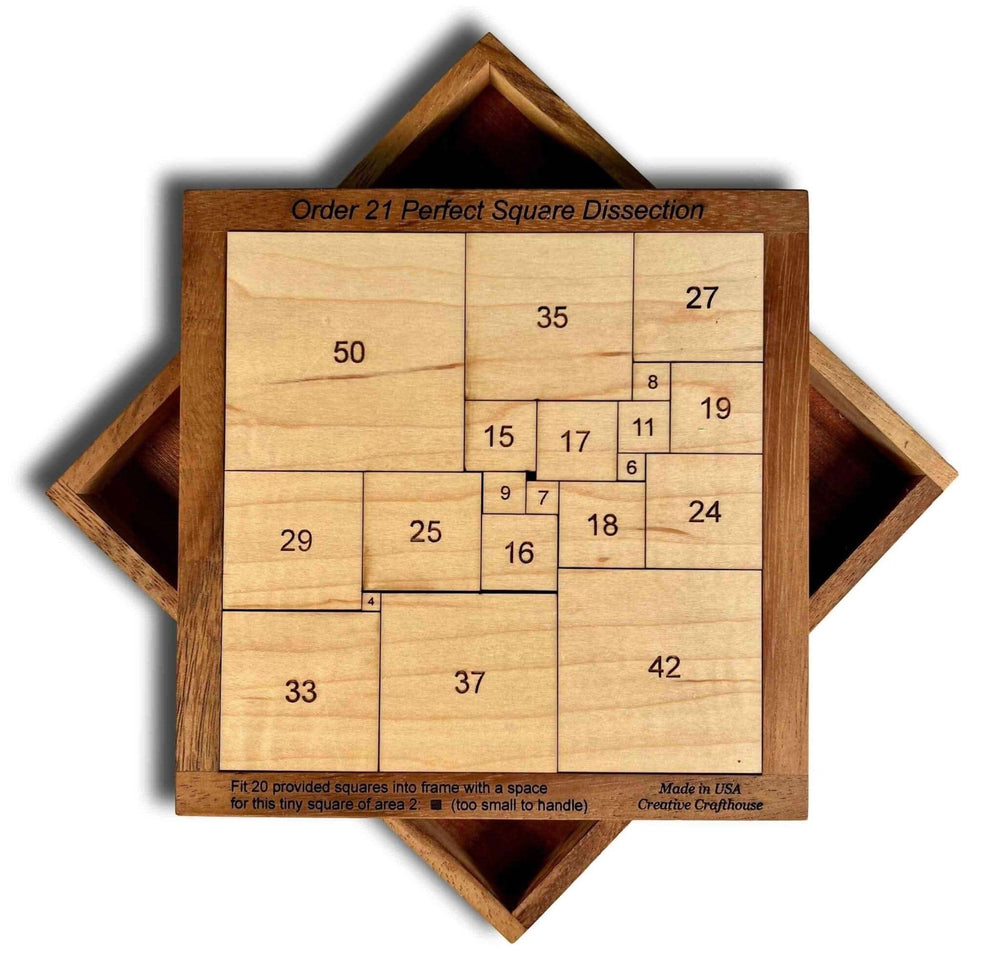
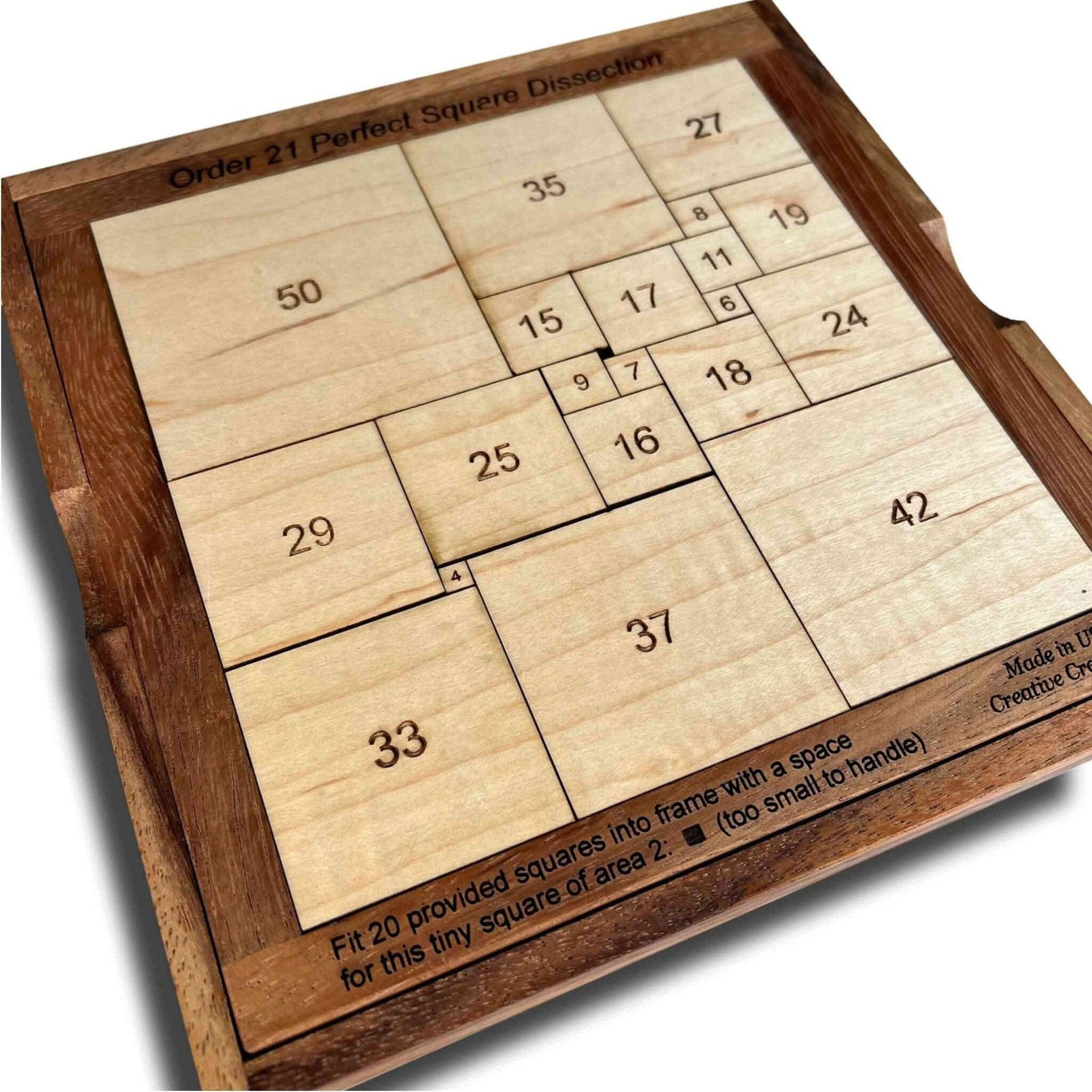
Discover the beauty of geometric dissections with the Order 21 Perfect Square Puzzle. Based on a groundbreaking mathematical discovery by A. J. W. Duijvestijn in 1978, this unique puzzle challenges you to arrange 20 wooden squares inside a frame, leaving an opening for the smallest 21st square, which is laser-engraved on the frame.
A Historic Mathematical Challenge
This puzzle represents the only known perfect square dissection of order 21, an important mathematical achievement. It provides an engaging way to explore geometry, logic, and spatial reasoning.
Premium Handcrafted Design
- Made from high-quality hardwood for durability and a beautiful finish.
- Laser-etched precision ensures each piece fits perfectly.
- Frame size: The completed puzzle forms a square with a side length of 112 units.
Who Will Love This Puzzle?
- Math Enthusiasts: A must-have for those fascinated by geometric dissections.
- Puzzle Lovers: A unique and rewarding challenge for serious solvers.
- Educators & Students: A hands-on tool for teaching math and logic in an interactive way.
Why Choose This Puzzle?
- Educational Value: Encourages critical thinking and problem-solving skills.
- Historical Significance: Based on the only known perfect square dissection of order 21.
- Great Gift Idea: Ideal for puzzle collectors, math teachers, and anyone who loves a challenge.
Made in the USA
Designed and handcrafted by Creative Crafthouse in Hudson, FL.
Personalize it!
Personalize it!
Add a personalized laser engraving to your puzzle or game.
- Perfect for gifts
- Business marketing opportunity
Payment & Security
Payment methods
Your payment information is processed securely. We do not store credit card details nor have access to your credit card information.
Product Specifications
- Dimensions
- 7.5 × 7.5 × 0.75
- Materials
- Wood
- Difficulty Level
- Hard
- Age Range
- Adults (18+ years), Teens (13-18 years)
- Piece Count
- 21
- What's Included
- Puzzle board (1), Puzzle pieces (21), Wood cover (1), Instructions and solution sheet (1)
- Gift Occasions
- Christmas, Birthday, Holidays
- Personalization Available
- Yes
- Puzzle Type
- Assembly, Dissection
- Category
- Brain Teaser Puzzle
- Availability
- In Stock
Personalize It!
Did you know we can add a custom laser engraving to every puzzle and game? Well, we can. Add a personal touch to your gift with a name or message.
Have you seen these yet?
Take a peek at some puzzles and games you might have missed.
| Facet | ||||
|---|---|---|---|---|
| By | ||||
| By | Creative Crafthouse | Creative Crafthouse | Creative Crafthouse | Creative Crafthouse |
| Price | ||||
| Price |
Regular price$33.95
|
Regular price$31.75
|
Regular price$26.49
|
Regular price$19.95
|
| Description | ||||
| Description | Challenging fun for both chess and non chess players. Handcrafted wood frame withcover and laser engraved wood pieces.Each puzzle comes... | Customizable Sudoku Board is a handcrafted wooden Sudoku game by Creative Crafthouse. Play one of the included preset puzzles or... | Falling Horses Game is a fast-paced wooden racing game for two players. Roll the die and race your horse to... | Penny Drop Game Medium is a handcrafted wooden dice game for family game nights and bar play. Two or more... |
